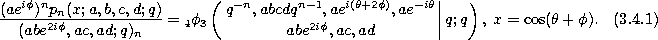
Definition.
Orthogonality.
If c = a and d = b then we have, if a and b are real and
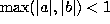or if 
and |a| < 1 :
where
with
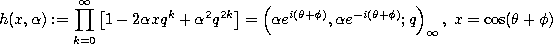
and
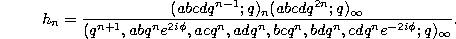
Recurrence relation.
where
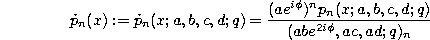
and
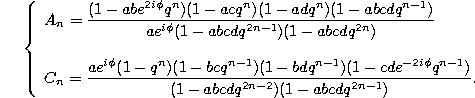
Normalized recurrence relation.
where
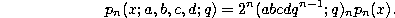
q-Difference equation.
where
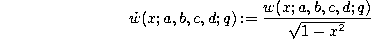
and
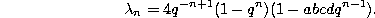
Forward shift operator.
or equivalently
Backward shift operator.
or equivalently
Rodrigues-type formula.
Generating functions.
Remark.
If we change q by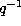 
we find
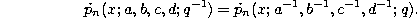
References. [31], [64], [193].
